Chapter One: An Acoustics Primer
7. What is amplitude? | Page 3
Power and Intensity
Acoustic energy is the amount of energy carried by a sound wave as it travels through a medium, such as air. We measure this energy in joules (J), which represent the work done when a force of one newton (N) moves something one meter. One newton, in turn, is the force needed to accelerate a one-kilogram object by one meter per second squared—like giving a gentle push that slowly speeds up a small book. Although these definitions sound abstract, they help explain how a sound wave compresses and expands the air around it, pushing and pulling on air molecules with minute forces measured in newtons. If the same sound is subjectively louder, it delivers more energy over time, meaning more joules are involved in driving the back-and-forth motion of the air molecules.
Power: The rate at which this energy is delivered—i.e., energy per unit time—is called power and is measured in watts (W).
1 watt = 1 joule (J) of work or energy transferred per second
A loud sound transfers more joules per second (more power) than a similar soft sound, increasing the energy that pushes and pulls on air molecules. As a result, a louder sound can be thought of as having a higher wattage than a softer version of the same sound.
Power does not change with distance (i.e. whether you view light from an 80-watt light bulb from the moon, or place your eyeball on it, its power is still 80 watts). A sound power level is a measurement of the total power generated by a sound source radiated in all directions.
Power is proportional (∝) to the square of the amplitude.
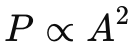
In other words, if you increase the amplitude of a sound wave, the power increases by the square of that change. When the amplitude of a sound wave doubles, the power increases by a factor of four (since 22=4). However, in real-world sounds with complicated waveforms, the amplitude fluctuates over time. In these cases, root-mean-square (RMS) amplitude is used to calculate an “average” power more accurately. (see previous page).
Technical Definitions Summarized
A unit of measurement for force is the newton (N), named after Sir Isaac Newton
1 newton will accelerate a 1-kilogram object by 1 meter per second squared
A unit of measurement for energy is the joule (J), named after James Prescott Joule
1 joule (J) = 1 newton (N) meter (m)
A unit of measurement for power is the watt (W), named after James Watt.
1 watt = 1 joule per second (J/s)
So, a watt (W) is a unit of power that measures the rate at which energy is transferred or used, a joule (J) measures the amount of energy being transferred when a force acts over a distance.
RMS power: The RMS measurement from the previous page is averaging the fluctuating amplitude over time, but is a somewhat controversial and often misused measurement for electrical and acoustic power. When used, it is typically measured into a resistive load or acoustic impedance for a fixed period of time. You may see published speaker or amplifier ratings in rms power in watts, meaning the maximum continuous average power they can either receive or generate via sine wave at minimal distortion into a resistive load of 4 or 8 ohms for a period of one minute without frying (resistance causes heat), as has been determined by government standards. Peak power differs from rms power in that it is the maximum non-averaged power a unit can output in a short burst, normally a much higher value, and some dishonest amp manufacturers used it deceptively implying it to be RMS power until the FTC put a stop to it. You may also see the specification PAPR, which is the peak-to-average-power-ratio.
Intensity: The power of the original sound source, along with distance of measurement from the sound source or the energy passing through a specific area (such as a square meter) perpendicular to the sound source, combine to form the intensity. Intensity (I) is referred to as a sound field quantity or root-power quantity (similar to rms on the preceding page).
Intensity is the power of a sound wave distributed over a unit area (such as a square meter), and it is proportional to the square of the wave's amplitude as well as power and energy.
As the surface area of the sound sphere expands, the amount of energy generated by the sound source is distributed over an exponentially increasing surface area. The amount of energy in any given square meter of the expanding sphere's surface decreases geometrically by the inverse square law, which states that the energy drops off by 
. Therefore acoustic energy twice the distance from the source is spread over four times the area and therefore has one-fourth the intensity. Simply put, relative intensity is the reciprocal of the change in distance squared or 
.
For acoustic sounds, the intensity values in W/m2 can be extremely small. In fact, while the threshold of discomfort is a whopping 1 W/m2, a vacuum cleaner (what's that?) at 1 meter produces about 0.00001 W/m2 .
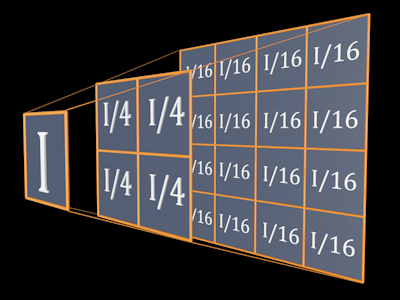
INVERSE SQUARE LAW
The inverse square law is extremely useful to remember in microphone placement, where even small changes in distance can have a significant impact on the resultant signal strength. The image above shows each doubling of distance exponentially reduces a sound's intensity at any given point, and that four times the distance will yield only 1/16th the intensity at a particular microphone's diaphragm location.
SOUND SPHERE MATH 101
You may recall from your grade-school math that the surface area of a sphere equals 4πr2, so as the radius of a sound sphere increases arithmetically, its surface area increases geometrically. The intensity of the source signal energy is distributed over the broadening surface area so that the 
Intensity is also proportional ∝ to the square of the amplitude.
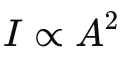
Sound Pressure Level (SPL)
Sound pressure level (or SPL), another sound field quantity, is an extremely useful measurement for acoustic study and recording and is frequently used to measure at the point of perception (i.e. the listener) or the placement of a microphone. It is a comparative measure of the relationship between a sound pressure at a certain distance (actually the rms of sound pressure amplitude) to a reference value for the threshold of human hearing, which is usually 0.00002 pascals (20 micropascals, or 20μPa), often described as the sound of a mosquito at 3 meters. SPL measurements without mention of distance, except for ambient sounds (for example a quiet room) are not often useful. SPL is normally expressed as a decibel value, and specifically labeled [dB SPL]. 0 dB SPL corresponds to the threshold of hearing. The correlation between sound pressure in pascals and dB SPL can range from 0.00002 Pa, which equals 0 dB SPL to 200 Pa which equals 140 dB SPL and beyond. The formulas for computing various forms of decibels are on following page.
While labeling is not always accurate, a sound quantity labeled "dB" without the SPL will usually refer to power or intensity, not sound pressure.
Intensity is proportional to the square of the amplitude. So if the amplitude of a sound is doubled, its intensity is quadrupled.
Power is also proportional to the square of the amplitude.
Therefore, power and intensity are proportional to each other.
We can finally say intensity (I) ∝ power (P) ∝ energy (E) ∝ amplitude (A)2
For a chart of common sounds relating dB SPL, amplitude as Pa, and intensity(I ) as W/m2, see this page.
Putting it all together
| Term | Property | Unit of Measurement |
|---|---|---|
| amplitude | Amplitude is the energy of a sound wave present, or the magnitude of maximum disturbance of the medium (air in our case), during one cycle of a periodic wave, known as peak deviation | pascals, or newtons per square meter (N/m2) |
| power | Power is the rate at which energy is being produced or used. In acoustics, this corresponds to the energy transmitted by a sound wave, which is proportional to the square of the amplitude of the sound wave over time | watt (also related is rms and SPL) |
| intensity | Intensity is the power present over an area (power per unit area), such as the outer surface of an expanding sound sphere. So from the top, intensity is the power transmitted through an area (such as a square meter), which is proportional to the square of the amplitude of the sound wave averaged over time. | watts per square meter (W/m2) |
Mini-Factoid: A chart of common sounds also follows, but it might be fun to mention here that a stun grenade at close distance, should you experience it, can produce a deafening 158-172 dB SPL.
